Mathematical models for wound healing: angiogenesis and wound contraction
Olmer van Rijn
Supervisor: Fred Vermolen
Site of the project:
Delft University of Technology
start of the project:
October 2009
In January 2010 the
Interim Thesis has been appeared
and a
presentation has been given.
The Master project has been finished in August 2010
by the completion of the
Masters Thesis
and a final presentation has been given.
For working address etc. we refer to our
alumnipage.
Summary of the master project:
Wound healing is a crucial process for the health and survival of an
organism. When a wound occurs, tiny blood vessels (capillaries) are cut and
blood enters the wound area. Blood clotting takes place and platelets start
releasing chemicals that signal the occurrence of the wound to the cells in
the undamaged surrounding tissue. These cells in the undamaged tissue start
proliferating and become mobile and move towards the wound area.
In cutaneous dermal wounds, fibroblasts move into the wound area so that the
dermis is restored. The fibroblasts produce collageneous tissue that is an
important constituent of the dermis. Besides restoration of the dermis, the
vascular system is restored. This process is commonly referred to as
angiogenesis. Once the dermis and vascular system have been restored, the
upper part of the wound closes. This lastmentioned process is referred to as
re-epithelialization and takes place by mobility and proliferation of
keratinocytes (epidermal cells). An intermediate effect is the contraction
of the wound, which is caused by the pulling mechanism of the fibroblasts.
Contraction of the wound is a crucial mechanism that minimizes the wound
area and hence reduces the number of contaminants that enter the wound
area. The pulling force gives rise to local tissue displacements and
strains. These strains influence the mobility, differentiation rate and
proliferation rates of the various cell types present in the wound area.
Mathematically speaking, the model that is used to simulate wound healing
involves the solution of viscoelastic equations to determine local strains
and stresses in the tissue. Further, the viscoelastic equations are
nonlinearly coupled with a set of nonlinear reaction-diffusion equations for
the various cell types, growth factors and vascular density. The equations
are solved using the finite-element method. A model for wound contraction,
as presented in the studies of Olsen (1995) and Javierre et al (2009) is
used and coupled with models for angiogenesis due to Maggelakis (2004) and
Gaffney et al (1999). The firstmentioned model for angiogenesis takes into
account the level of oxygen, whereas the
second model for angiogenesis takes into account the capillary tip density
and endothelial cell density. This lastmentioned model involves
cross-diffusion and source terms that are inspired from probability
arguments.
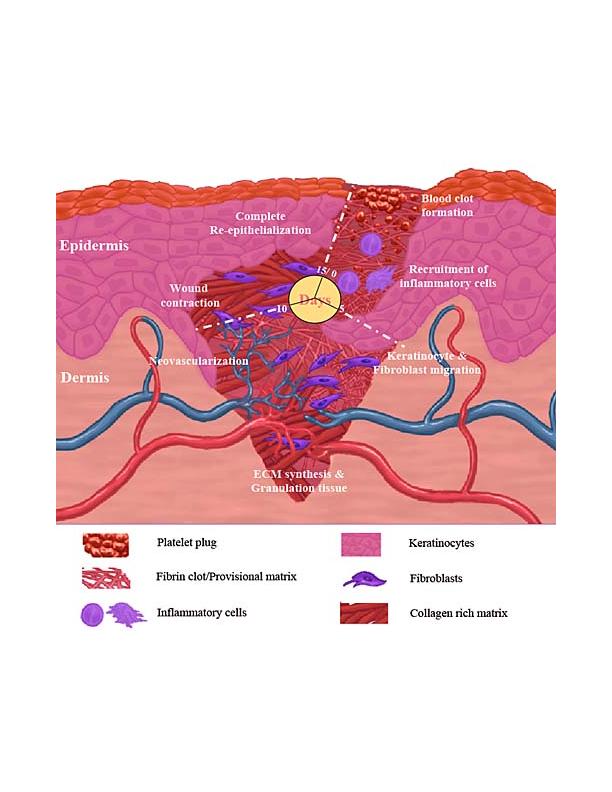
The structure of the dermis with a wound

Contact information:
Kees
Vuik

Back to the
home page
or the
Master students page of Kees Vuik

![]()
![]()