Numerical treatment of stochastic control problems
by Fourier-cosine series expansions
The dike height problem
Marjon Ruijter
Supervisor: Kees Oosterlee
Site of the project:
Delft University of Technology
start of the project:
November 2009
The Master project has been finished in September 2010
by the completion of the
Masters Thesis
and a final presentation
has been given.
For working address etc. we refer to our
alumnipage.
Summary of the master project:
In financial markets traders deal in stocks and options, such as the well-known call and put options. An
option is a contract between a buyer and a seller that gives the buyer the right to buy or sell a particular stock under prescribed conditions. In this turbulent trading world, questions about a `fair' option
price and the hedging of risks arise. In the recent decades financial mathematics has developed fast to
contribute to this theory and to improve the pricing methods.
In economical, but also in personal or societal contexts, one may face options in the sense of real `choices'.
For example, should one build a new factory now or later. These options are called real options and can
be related to the financial options.
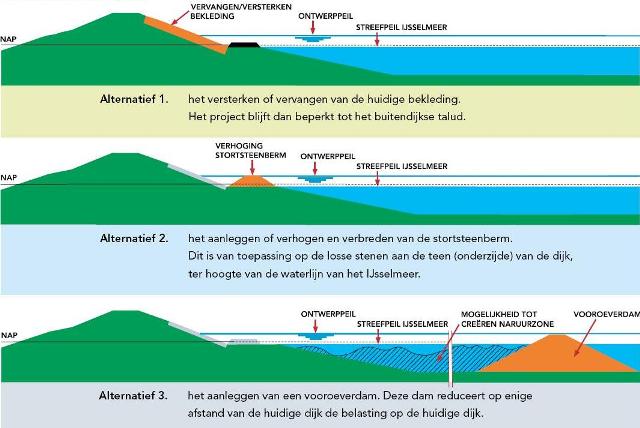
Alternatives for a stronger dike

Dike height increase

Contact information:
Kees
Vuik

Back to the
home page
or the
Master students page of Kees Vuik


![]()
![]()